Experiments with the blind spot and filling in

How to draw your blind spot and the blood vessels that obscure vision

The Figure below is a photograph of the author's retina, seen from the front. From where they enter the eyeball, the blood vessels spread out to cover the surface of the retina in the way giant spiders might straddle each eye in a science fiction film. The blind spot is visible on the left side, as the body of the spider, from which the legs radiate out.

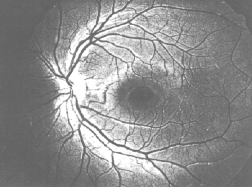
Under special conditions it is possible to make the blind spot and blood vessels visible[1]. In  the method which is most effective, you take a strong penlight with a small, concentrated beam, and hold it very close to the side of the eye, so that its light creates a small bright spot on the white of the eye. This strong light traverses the cornea and creates a reddish glow on the inside of the eyeball. Since this glow is located to the side of the pupil, that is, at a spot inside the eyeball where usually no light comes from, the shadows of the retinal blood vessels that are cast by this glow are at an abnormal position and become noticeable, particularly when the flashlight is moved slightly[2].

What you see is quite similar to what is seen in Figure above. There is a large knot of vessels, corresponding to the blind spot, on the side away from the nose and slightly below the horizontal midline. Looking straight ahead however, that is, in central vision, there are not many vessels. This because at the very center of the visual field there is a region called the fovea where the photoreceptors are most concentrated and where vision is keenest. Presumably so that foveal vision can be as acute as possible, the web of blood vessels carefully skirts around this spot. The spot is called fovea ('depression', in latin) because under the microscope it appears as a slight dimple in the surface of the retina.

A simple way of showing that you actually see nothing in the region of the blind spot is the following. You close the left eye, for example, and look with the right eye at your left thumb, held at arm's length.  (It is important to get the sides right, since the blind spot is situated asymetrically, on the nasal side of each retina, that is, the blind spot of the right eye is on the right of the line of sight and the blind spot of the left eye is on the left of the line of sight). Next to your left thumb you hold your right thumb, which you gradually move away from the left thumb, towards the right. At a separation between your thumbs of about 6 - 10 inches (corresponding to an angle at the eye of about 10 - 15 degrees), while you continue looking at the stationary left thumb, your right thumb will disappear from view (of course it will reappear if you look at it directly). By moving your thumb slightly, you can ascertain that the blind spot is not situated exactly on the horizontal midline, but about 2-3 degrees below it. You can also see that it is surprisingly large: its diameter is about 5-6 degrees horizontally and 7-8 degrees vertically[3]: about the side of 12 full moons[4] set next to each other. This means that the blind spot can be made to devour a lemon or small orange held at arm's length.

Given the size of the blind spot, it is surprising that it such great observers as Aristotle, Galen, and Al Hazen did not discuss it, and that it only became known after Mariotte (1668) caused a sensation with it at the Royal Society of London, and after King Charles II supposedly amused himself by visually decapitating his courtisans[5].

Helmholtz[6] gives an excellent method for drawing your own blind spot. You close one eye (say the left) and you look at a fixation point marked on a piece of paper. You localize the region of the blind spot to the right (when looking with the right eye) of the fixated point, and with a pencil, you blacken the paper over all the area for which the tip of the pencil remains invisible. As soon as the tip of the pencil comes out of the blind spot, you stop marking. By proceding in this way, and keeping the eye completely still (this is difficult!), it is possible to draw the vertically extended patch corresponding to the blind spot, and even to begin to draw the stumps of the two blood vessels that leave the spot at the top and at the bottom. The technique is illustrated below, on the left.

 

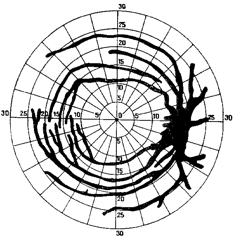
The Figure on the right (from Weekers, 1945) is the result of performing just the same kind of mapping of the visual field, but with more precision, and in conditions of weak illumination so we can descern not only regions that are completely blind, like the blind spot, but also regions that are less sensitive to light because they are obscured by blood vessels. The figure is surprising, because it demonstrates both how vast the regions are that are obscured by the web of retinal blood vessels, and also the extent to which we are ignorant of these vessels. Why is it that we do not ordinarily notice this vast web of vessels casting shadows all over the visual field? Why don't we see the world as though we were peering out from behind the blood vessels? Why dont we see the blind spot like the body of a giant spider, from which the legs radiate out? The absence of blood vessels at the fovea explains why vision is not deteriorated in the center of the visual field, but why does one not see a large empty blob corresponding to the blind spot, off to the side of each eye? Why does one need to resort to artificial methods like Helmholtz's method described above, in order to become aware of the large gaps in our visual field

White eye vs. red eye in flash photography

Another interesting thing about the blind spot is that you can actually see it from outside someone's eye. To understand this, consider the well-known effect of 'red-eyeÓ when you take a flash photograph of someone. The reason people's eyes look red is that the light from the flash travels into the eye, gets reflected by the retina, and comes out again along the same path that it went in. When the camera lens is positioned very close to the flash, as is usually the case in amateur photography, this reflection is recorded on the film. Now, since the retina is covered with blood vessels and photoreceptors, it absorbs most of the light, and only reddish light comes back out. But at the blind spot, nerve fibers bunch together to get out of the eye. There are no photoreceptors, no light absorption, and, so most of the light landing on the blind spot simply gets reflected. This means that if you were to take a flash photograph of someone who had placed their eyes with their blind spot exactly at the position of the flash, the photograph should show 'white-eyeÓ instead of 'red-eyeÓ. And indeed this is what happens, as can be seen in the picture below


 


Red eye and white eye! My son and daughter have placed their eyes in such a manner that their left eye's blind spot is positioned on the flash. For this reason a bright white spot appears on their left pupil, whereas their right pupil shows the usual, weaker, red-eye effect deriving from reflection of the flash off a different portion of the retina. The chances of this occurring unintentionally are small, which explains why one rarely sees 'white-eyeÓ in photographs.

 

 

The disappearing lollipop trick

Close your left eye and look at the cross on the left. Gradually move the book closer and closer to your eye (be careful: make sure you have the LEFT eye closed and the right eye open). Suddenly, at a distance of about  15 cm, the lollipop on the right will disappear, only the stick will remain[7].

 

 

If you redo the disappearing lolipop trick described above, but with a two-handled lolipop as shown below, something curious happens. Now although the heart of the lolipop disappears as before, the handles join up to make a single line that appears to cross the blind spot.

 

 

 

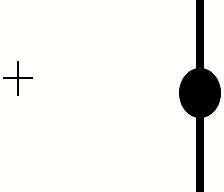
Another interesting test of the blind spot's intelligence is flowery wall-paper. If the flowers are fairly small, so that they form an overall texture, you will find that you don't have the impression that there is anything missing in the blind spot. Admittedly it's hard to tell exactly what is present there, but you certainly don't have the impression that there is an interruption of the surrounding flower-texture.

On the other hand if the flowers are fairly big, so that only a single flower will fit into the blind spot, then you definitely have the impression that there is a missing flower.

A great debate has arisen in the vision scientist community about experiments like this. Many vision scientists suppose that the phenomena must be explained by an active filling-in mechanism that takes the visual image and actually 'paints inÓ the information that is missing by starting at the boundaries of the blind spot and spreading inwards[8].

Indeed vision scientists have additional reasons for thinking that there should be such a spreading activation mechanism. It seems that the perception of surface color and surface brightness depend critically on the properties of the edges and boundaries that delimit the colors.

A classic example is the so-called Cornsweet-Craik-O'Brien illusion you see in the following Figure.

 

Cornsweet-Craik-O'Brian illusion. Even though the left half looks darker, in fact the two halves of the square are the same lightness except at the central boundary. The boundary is constructed in a special way—it has a luminance profile as shown in the graph under the figure: just as you reach the boundary coming from the left, the lightness decreases slightly, then jumps up to a slightly higher value, and then goes down to the same value that it was at the left of the Figure.

You have the impression of seeing two surfaces of different lightness. But in fact they are equally bright. You can convince yourself of this by putting a pencil in such a way as to hide the boundary between the surfaces. The explanation is that the boundary between the two surfaces has a special profile that induces the effect.

Here are two more examples showing that the perception of the color and lightness of surfaces depends on the presence of abrupt changes in luminance. In these cases illusory bright surfaces seem to be generated by nearby abutting lines.

These examples are compatible with the existence of neural brightness-filling mechanisms that work inwards from luminance discontinuities. The filling-in of the blind spot may be another manifestation of such a mechanism.

 

           

On the left, the Ehrenstein illusion: The abutting lines create the effect of a whiter-than-white disk floating above the background. On the right, the Kanizsa triangle: The white triangle formed by the pacmen and the interrupted black lines seems whiter than the white of the page, and seems to have a real boundary, whereas in fact there is none.

Other scientists on the other hand have a different interpretation of all these effects. Consider the following set of dogs. Clearly the dog behind the wall is much longer than his friends. Interestingly however you don't have the impression of seeing the whole length of the partially hidden dog. You somehow know or sense that he is longer, but you don't have the feeling of his belly being actually painted in. The brain seems to somehow assume that the rest of the dog is there, behind the wall, without actually recreating it. This phenomenon is called 'amodal completion', and seems to be a less visually 'present' form of filling in than what we observe in the virtual contours and bright areas of the Cornsweet-Craik-O'Brien, the Ehrenstein and the Kanizsa illusions.

 

 

Amodal completion of a dog. Even though the middle dog's tummy is hidden, one has the almost palpable impression that it really is there.

 

Could it be that what happens in the blind spot is more like amodal completion than true active 'painting in' of missing information? The brain would have to do less work that way, since all that is necessary is that it should assume that there is the same kind of stuff in the blind spot as nearby, and no active painting-in has to occur. This also makes sense to the extent that we can't really see very accurately what is in peripheral vision anyway.

The "homunculus" objection doesn't apply so much to this type of interpretation of filling-in: Under this 'assuming it is there' hypothesis, no internal 'picture' of the missing parts of the scene are created for some internal homunculus to contemplate, since the information the observer has about the missing parts of the scene is more a form of knowledge than a real visual 'presentation'. But while this seems to square quite well with the subjective impression you get in the case of amodal completion, there are cases like the virtual contours or the brightness filling-in, where the feeling is somehow more 'real' or 'visual'.

Despite homuncular doubts, the idea of filling-in seems eminently reasonable, and can be found in virtually every textbook on vision. Clearly filling-in, be it the active type or the amodal type, exists in the sense shown in the illustrations above.


[1]Purkinje gave three methods,  described briefly in Le Grand, 1956, Vol 3, p. 159.

[2]There is an interesting historical fact concerning this method of seeing the vascular scotoma. Up until the end of the 19th Century the nature of the retinal photoreceptors was not known, nor was the exact location where the transduction of light into nervous impulses occurred: was it the superficial or the back layers of the retina, or was it at the choroid (XXX check not choroid membrane) itself? Using a variation of the trans-scleral illumination method described here, the WŸrzburg anatomist Heinrich MŸller in the 1850's measured the amount of shadow motion that occurred when he moved the light source, and deduced that the light sensitive regions must be at the back layers of the retina, furthest from the incoming light (cf. Polyak 1957,  p. 52).

[3]These numbers are from Dubois-Poulsen et al. (1952), who has compiled the location of the blind spot over numerous observers. Its size is determined principally by the size of the region without photoreceptors at the head of the optic nerve (about 5 degrees in diameter). However the blind region is rendered larger than this by the presence of the knot of retinal blood vessels, which extend somewhat outside the zone of the head of the optic nerve. In this region the eye is not completely blind, because the vessels are not opaque. The size of the effectively blind area thus depends on what can be seen through the blood vessels: on the stimulus color, contrast and on lighting conditions. Furthermore, the extent of the blind zone will also be influenced by anything that affects the diameter of the blood vessels: the effect of medicines, mechanical pressure on the eye, vascular and emotional state, and on the time of day: it seems that the blind spot is 2 to 5 degrees larger in the morning than in the evening. cf. Dubois-Poulsen, 1952, cited by Le Grand, 1956, Vol 3, p 157.

[4]This comparison is due to Helmholtz, according to Le Grand, 1956, Vol 3, p. 157.

[5]according to Le Grand, 1956, Vol 3, p. 155.

[6]In Chapter 6 we will speak in more detail of Helmholtz, whose Handbook of Physiological Optics is still many vision workers' bedside reading.

[7] To make your thumb disappear, put your two thumbs next to each other and stretch out your arms in front of you. Close your left eye and look at your left thumb with your right eye (admittedly this is a bit confusing!). Gradually separate your thumbs still looking at the left thumb with your right eye. Presto! Suddenly, when your thumbs are about 15-20 cm apart, the right thumb disappears. (It helps to hold the right thumb about 2 cm lower than the left; Remember you are looking at your left thumb: the right thumb disappears in periphery, in indirect vision).

[8] Paradiso & Nakayama (1991) have actually studied the dynamics of the filling-in process as it proceeds from the edges of a figure into the middle. Moreover, both for brightness and textural filling-in psychophysical investigations have unveiled a temporal dynamics as of a gradual filling-in process, and, in the case of brightness, this has been measured as having a speed of the order of 110/150 degrees per second. c.f. Rossi & Paradiso 2003. Surface Completion: Psychophysical and Neurophysiological Studies of Brightness, in Pessoa & De Weerd, eds., 59- 80. Spillman & De Weerd 2003. Mechanisms of Surface Completion: Perceptual Filling-In of Texture, in Pessoa & De Weerd, eds., 81- 105. For a philosophical discussion in relation to consciousness see Erik Myin & Lars De Nul (XXX) Filling In, in Bayne, Cleeremans & Wilken eds. The Oxford Companion to Consciousness.